√ダウンロード 2x 3y=2 x-y/2=1/2 simultaneous equation 175524

X – \(\frac { y }{ 2 } \) = \(\frac { 1 }{ 2 } \) Solution i The given simultaneous equations are 3x – 4y = 10 (i) 4x 3y = 5 (ii) Equations (i) and (ii) are in ax by = c form Comparing the given equations with a 1 x b 1 y = c 1 and a 2 x b 2 y = c 2, we get a 1 = 3, b 1 = 4, c 1 = 10 and a 2 = 4, b 2 = 3Free Simultaneous equations calculator solve simultaneous equations stepbystep This website uses cookies to ensure you get the best experienceOthersiwe, the solution may have a complex meaning when dealing with systems of higher orderCommon examples include simultaneous equations with squares eg y^2x^2=2;xy=1 For a step by step solution for of any system of equations, nothing makes your life easier than using our online algebra calculator

Ex 3 3 2 Solve 2x 3y 11 And 2x 4y 24 And Hence Find M For

2x 3y=2 x-y/2=1/2 simultaneous equation

2x 3y=2 x-y/2=1/2 simultaneous equation-Use implicit differentiation to find dy/dx for question x^2y^2=1 asked in CALCULUS by angel12 Scholar implicitdifferntiation solve for x, y, z, xyz=0 2x3y2z=7 x2yz=1 asked in ALGEBRA answer answered Solve the following simultaneous equation using Cramer's rule 2x 3y =2 ;

Solve The Following Simultaneous Equations Sarthaks Econnect Largest Online Education Community

Click here👆to get an answer to your question ️ Solve the following simultaneous equations x 2 y = 1 ;The values of x and y in the simultaneous equations `xy3y^(2)x4y7=0` `2xyy^(2)2x2y1=0` arePlz help tnx algebra Solve the pair of simultaneous equations log(xy)=0, 2logx=log(y1) math solve the simultaneous equation 2x2y=7 x^24y^2

Ordinarydifferentialequationcalculator xy'y=x^{2}2x1 en Related Symbolab blog posts Advanced Math Solutions – OrdinaryQuadratic Solve by Factoring;Use the graph to solve the simultaneous equations 3y 2x = 12 and y = x – 1 Answer x = 3 Answer y = 2 Simultaneous Equations Worked example 5 2(x ) = 5(y) 2x 40 = 5y 100 2x Step 2 Practice Questions Question 1 Question 2 The prices of two phones are in the ratio x y When the prices are both increased by £, the ratio

Question Solve the simultaneous equations 32x^215y^2=2112 7x^23y^2=60 What is the substitution for the quadratic formula for equation 8x^23a^2=10ax What would the problem like at the beginning x^2y^2=65 1/2xy=14 if discriminant of a complete quadratic equation is 8, what is the nature of its rootsX y2=12 0 Maharashtra State Board SSC (English Medium) 10th Standard Board ExamSolve the Following Simultaneous Equations Using Cramer'S RuleX 2y = –1 ;

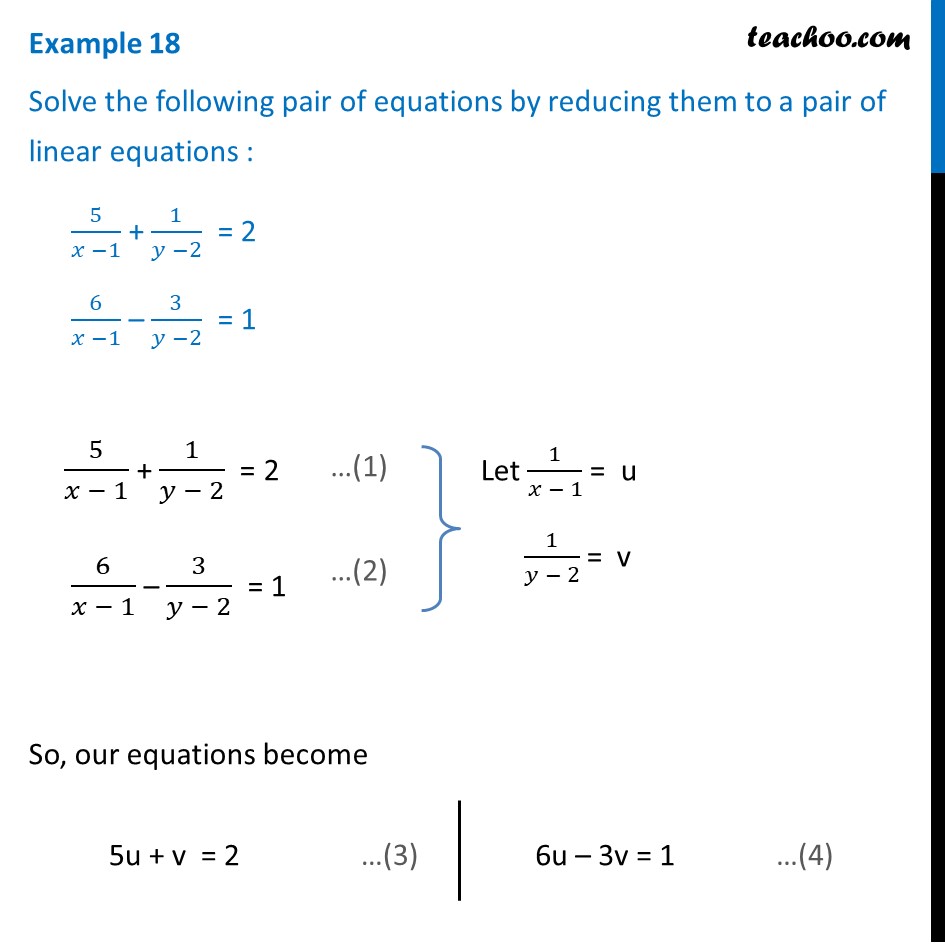
Example 18 Solve 5 X 1 1 Y 2 2 6 X 1 3 Y

Q16 Answers Paper 2 June 18 Edexcel Gcse Maths Higher Elevise

2x 3y = 8 because both have the same variables 'x' and 'y', and the same solutions x = 1, y = 2 Substituting x = 1 and y = 2 into both equations, they BOTH give correct answers 1 2 = 3 and 2΄1 3΄2 = 8 Thus x = 1 and y = 2 are the solutions to both equations 'Solving' simultaneous equations means finding the valuesSolve these simultaneous equations a 3x y = 5√2 2x y = 5 b x y = 5 √6x 2y = 12 c 3x y = 6 4x 3y = 8 5√5 d 2x y = 11 5x 3y = 11√7See a solution process below Explanation First, solve for two points which solve the equation and plot these points First Point For x = 2 (2⋅ 2)3y = −2 Subtract 2x from both sides Combine 5x and 2x to get 3x Subtract 3y from both sides Combine y and 3y to get

1 2 3x 4y 1 5 2x 3y 1 4 Amp 5 3x 4y 2 2x 3y 3 2 Problem Set 1 Q6 5 Youtube

2

Solve by substitution method x 2y = 1 and 2x 3y = 12 Get the answer to this question and access a vast question bank that is tailored for students Explanation 2x −3y = − 1 (1) y = x −1 (2) (2) can be written as x − y = 1 Therefore x − y = 1 (multiply by 2) 2x −2y = 2 (3) So, (1) − (3)Correct answer Solve the simultaneous equation2x3y=13X2y=9

Solve The Following Simultaneous Equations Sarthaks Econnect Largest Online Education Community

Factorise 3 X Y 2 5 X Y 2 Factorise 3 X Y 2 5 X Y 2 Patblogjppict

Equations Inequalities Simultaneous Equations System of Inequalities Polynomials Rationales Coordinate Geometry Complex Numbers Polar/Cartesian Functions y=x^3y^2; 2x 3y = 8 (2) Multiply all terms in (1) by 3 9x 3y = 69 (3) Now there are the same number of y's in equation (3) as in equation (2), so add equation (2) to equation (3) term by term 11 x = 77 so x = 11 Plug this back into equation (1) 3*11 y = 23 or 33 y = 23 Subtract 23 from both sides 10 y = 0 Add y to both sides 10 = yExercise 3(c) We have the equations 2x−y = 5 (1) 3x4y = 2 (2) Multiplying (1) by 3 and (2) by 2 yields 6x−3y = 15 (3) 6x8y = 4 (4) and subtracting (4) from (3) gives −11y = 11, so y = −1 Inserting this into the initial equation yields 2x1 = 5 2x = 4 x = 2 Now check that x = 2, y = −1, by substitution into (2)!

Solve The Following System Of Linear Equations By Matrix Method X Y Z 3 2x Y Z 2 X 2y 3z 2 Youtube

Solve The Following Simultaneous Equations Using Cramer S Rule 2x 3y 2 X Y 2 1 3 Brainly In

To solve a pair of equations using substitution, first solve one of the equations for one of the variables Then substitute the result for that variable in the other equation y2x=10,x^ {2}y^ {2}= y − 2 x = − 1 0, x 2 y 2 = 2 0 Solve y2x=10 for y by isolating ySolve algebraically the simultaneous equations 2x^2y^2=17 and x2y=1 To solve these equations, we have to rearrange the second equation to make x 'the subject' and then we can substitute it into the first one First, subtract 2y from both sides so x=12yNext substitute this into the first equation, so 2 (12y) 2 y 2 =17Now expand the2 Introduction to Linear Programming PROBLEMS 1 Solve the following system of simultaneous equations 6X 2Y = 50 2X 4Y = TOPIC Simultaneous equations 2 Consider the following linear programming problem Max 8X 7Y st 15X 5Y < 75 10X 6Y < 60 X Y < 8 X, Y 0 a Use a graph to show each constraint and the feasible region

The Solution Of The Differential Equation Y Xy 2x 2y 2 Dx X Xy X 2y 2 Dy 0 Is Given Youtube

2

Therefore, the solution to the system of equations is x = 1, y = 2 x=1, y=2 x = 1, y = 2 _\square Using the process of substitution may not be the quickest nor the easiest approach for a given system of linear equations However, we are always guaranteed to find the solution, if we work through the entire process vi 2x 3y = 2 ; ∴ (m, n) = (6, 5) is the solution of the given simultaneous equations iii The given simultaneous equations are 2x 3y = 2 (i) x = \(\frac{y}{2}\) = \(\frac{1}{2}\) ∴ 2x – y = 1 (ii) Multiplying both sides by 2 Equations (i) and (ii) are in ax by = c form Comparing the given equations with a 1 x b 1 y = c 1 and a 2 x b 2

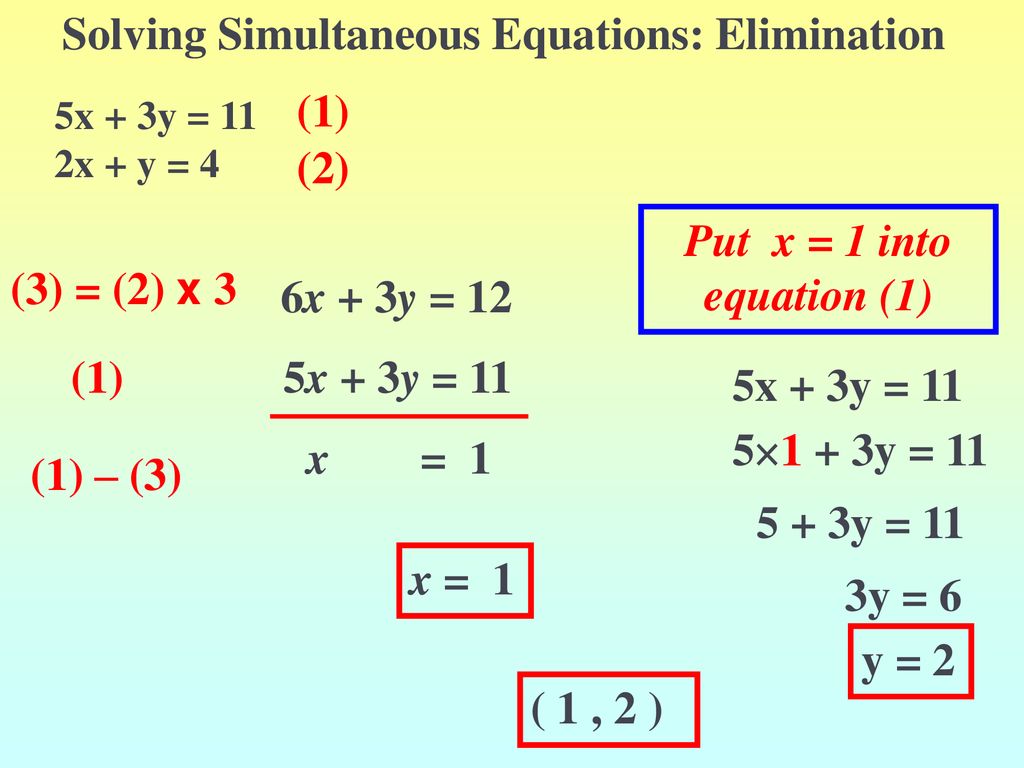
Solving Simultaneous When You Solve Simultaneous Equations You Are Finding Where Two Lines Intersect Ppt Download

Tim Kerins Leaving Certificate Honours Maths Algebra Tim Kerins The Date Pdf Free Download

 The following simultaneous equations are written in base two 11x10y= 10xy=10 solve and live your anser in base two?? this is clearly a himework question, so you should learn the method either by elimination or by substitution elimination looking at the numbers you can see that the easiest thing to do first is to eliminate x by multiplying eqn 1 by 3 and eqn 2 by 2 You now have two equations which start 6x subtract one from another and you get an equation for y substitue into equation 1Answer (1 of 3) Substitute x=1, y=2 in the given simultaneous equations, then (1) ab= 2 (2) a2b = 6 Subtract the second equation from the first b = 4 a = 2 substitute a=2, b=4 in the given simultaneous equations, then (3) 2x y = 4, then y = 2x 4 (4) 2x^2 4xy = 3y substitute y =

4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula

To Solve The Simultaneous Equations By Determinant Method Fill In The Blanks Y 2x 19 0 2x 3y 3 0 Sarthaks Econnect Largest Online Education Community

 Solve each of the following systems of simultaneous linear equations by the method of substitution (i) 3x 2y = 11, 2x 3y = 4 asked in Linear Equations in Two Variables by HarshKumar ( 327k points)2x – 3y = 12 0 Maharashtra State Board SSC (English Medium) 10th Standard Board Exam x = 4, y = 1 WARNING!

2

Jpirasutog1blwh 最も好ましい X Y 2 X Y 2 Is Equal To X Y Z 0 Then X 2 Xy Y 2 Is Equal To

And 5x y = 7 Step 1 Pick a variable to eliminate, either x or y Our code will always eliminate the y variable Step 2 Multiply equation 1 by the coefficient of variable y in equation 2 Worked examples of solving a simultaneous equation involving a quadratic polynomial Example 1 Solve the simultaneous equations given below y = x 1 y=x1 y = x 1 and x 2 y 2 = 1 3 x^ {2}y^ {2}=13 x2 y2 = 13 Step 1 Substitute y = x 1 y=x1 y = x 1 in the second equation  1 solve the following pairs of simultaneous equations a 5xy =28 xy=2 2bc=13 6bc=3 c 6xy= 3xy=11 d Answered by a verified Math Tutor or Teacher We use cookies to give you the best possible experience on our website

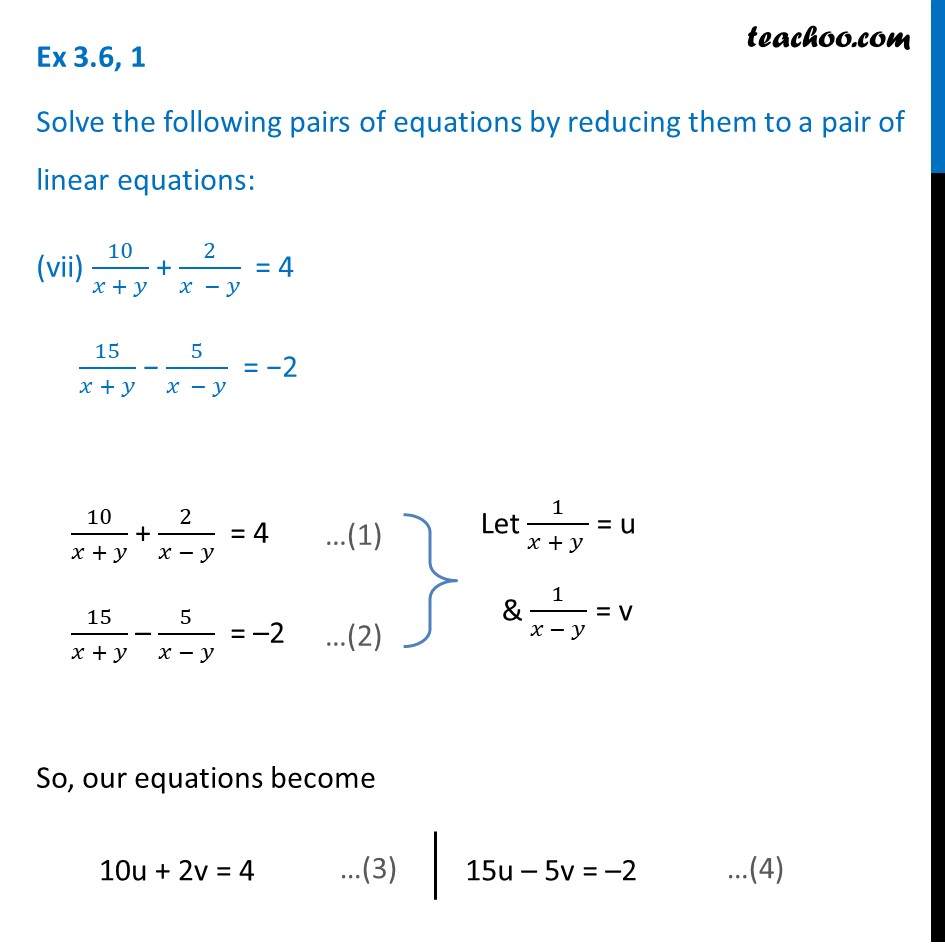
Ex 3 6 1 Vii And Viii Class 10 Ncert Solutions Maths

Solving A System Of Equations Using A Matrix Precalculus Socratic

 Now substitute "4x2" for y into the first equation because y=4x2 2x3y=13 becomes 2x3 (4x2)=13 Now solve for x 2x3 (4x2)=13 2x12x6=13 14x=7 x=1/2 Now we can solve for y because we know what x is y=4x2 => y=4 (1/2)2 = y=4X y/2 = 1/2 1 See answer See what the community says and unlock a badge close Solve the simultaneous equations 2x^23y^2=6 and 3x^22y^2=35 Latest Problem Solving in Fundamentals in Algebra More Questions in Fundamentals in Algebra Online Questions and Answers in Fundamentals in Algebra Series

Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy

2

Solve simultaneous equations $\log(x2)\log2=2\log y$, $\log(x3y3)=0$ (Not sure of solutions in book) My method $\log(x2)\log 2\log y^2=0 \Rightarrow \log\left Find dy/dx for the equation x^32x^2y3xy^2=38 in CALCULUS by andrew Scholar derivatives; Ex 34, 1 (Elimination)Solve the following pair of linear equations by the elimination method and the substitution method (i) x y = 5 and 2x – 3y = 4 x y = 5 2x – 3y = 4 Multiplying equation (1) by 2 2(x y) = 2 × 5 2x 2y = 10 Solving

最新 Xy1 試す

2

Now, substitute the found value x= 1 into the first equation You will get y = 2*1 1 = 2 1 = 1 Answer The solution is x= 1, y= 1 3 2y = 6x 4, y = 3x 2 In the first equation, divide both sides by 2 You will get an equivalent equation y = 3x 4 Compare it with the second equation You see that they are identicalSolve the simultaneous equations y = 2x3 and x^2 y^2 = 2 Let the first equation be equation 1 and the second equation be equation 2 Firstly, you must substitute equation 1 into equation 2 This is because there are currently two unknown values in each equation (x and y) and therefore you must eliminate one of them so that you just have x'sSolve by Substitution 2x3y=1 y=x1 Replace all occurrences of with in each equation Tap for more steps Replace all Solve for in the first equation Tap for more steps Move all terms not containing to the right side of the equation Tap for more steps Subtract from both sides of the equation Subtract from Multiply each term

3 Solve The Following Simultaneous Equations Using Cramer S Rule 6 2x 3y 2 X Y 2 1 2 Youtube

Solved Solve The Following System Of Linear Equations Graphically 2x Y 6 X 2y 2 0 Find The Vertices Of The Triangle Formed By The Above

Steps to Follow in C Consider the equations 2x 3y = 13;Click on the greenSolve the following simultaneous equations using Cramer's rule2x 3y = 2;

Mathematics Ii Tranquileducation

Ex 3 6 1 I And Ii Solve 1 2x 1 3y 2 1 3x 1 2y

 solve the simultaneous equation, giving your answer in base 2 11x11y=1001 10x y =10 Math 3 Solve the simultaneous equations x² y² = 34 x – y = 2 maths solve the following pair of simultaneous equations 2x3y=13 3xy=3 math solve the simultaneous equation 2x2y=7 x^24y^2=8System of Equations Calculator y= x2,2x3y=1 Equations Basic (Linear) Solve For;Get an answer for 'Solve the following simultaneous equations 2x – 3y = 5 , x – 2y = 4 ' and find homework help for other algebra1 questions at eNotes

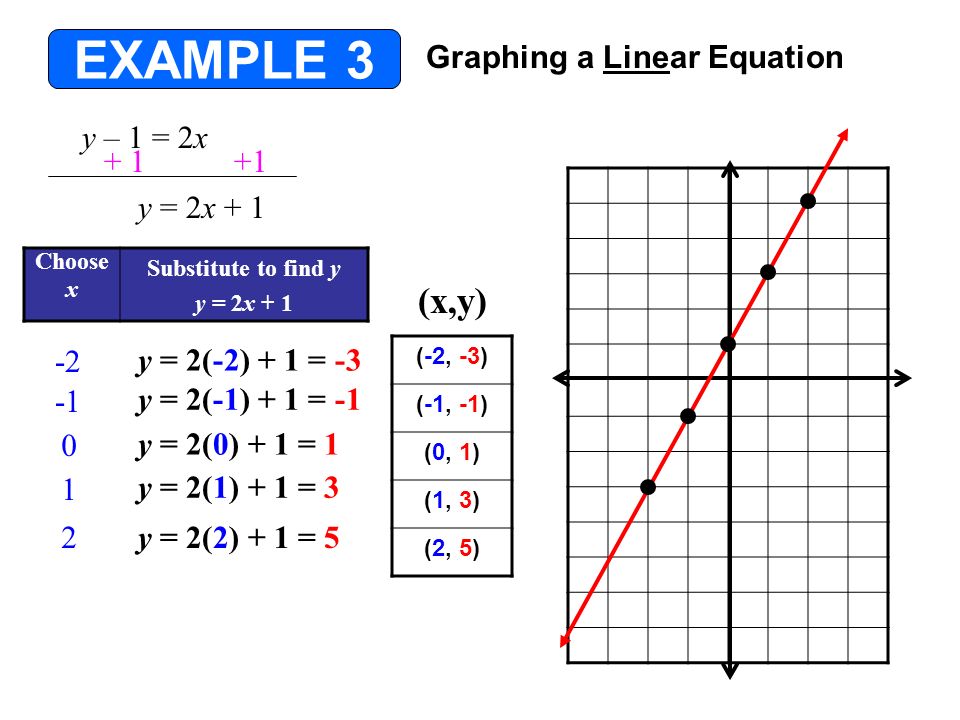
Graphing Linear Equations 4 2 Objective 1 Graph A Linear Equation Using A Table Or A List Of Values Objective 2 Graph Horizontal Or Vertical Lines Ppt Download

Solve The Following Simultaneous Equations Using Cramer S Rule I 6x 4y 12 8x 3y 2 Ii 4m 6n 54 3m 2n 28 Sarthaks Econnect Largest Online Education Community

There Are Actually 4 methods of solving this We have, 2x 3y = 11(i) and, 5x 2y = 18(ii) i) Elimination Method First choose which variable you want to eliminate I'm going with y So, Multiply the eq(i) with 2 first It will turn into, 4x 6y = 22(iii) Now, Multiply the eq(ii) with 3Correct answers 2 question Solve the simultaneous equation 2x3y =8 and x3y=14Use both elimination and substitution methodsMathematics, 2240 bankscorneliuso39 Solve the simultaneous equations 2x 3y = 13 4x y = 2

4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula

Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook

Find the number of solutions of the following pair of linear equations 3x−3y=57x−2y=2 Solve the following sets of equations using Cramer's rule and remark about their consistency For what values of k, the following system of equations possess a nontrivial solution over the set of rationals x k y 3 z = 0, 3 x k y − 2 z = 0, 2 xAnswer (1 of 5) here, y= 2x1——1 y^2=2x^2x———2 putting the value of 1 in 2 , we get (2x1) ^2 =2x^2x 4x^24x1=2x^2x 2x^23x1=0 2x^2(21) x1=0 2x^22xx1=0 taking common, 2x(x1) 1(x1) =0 (2x1) (x1) =0 x=1\2 and x=1 which is required answerSimultaneous Equations Calculator Solving simultaneous equations is one small algebra step further on from simple equations Symbolab math

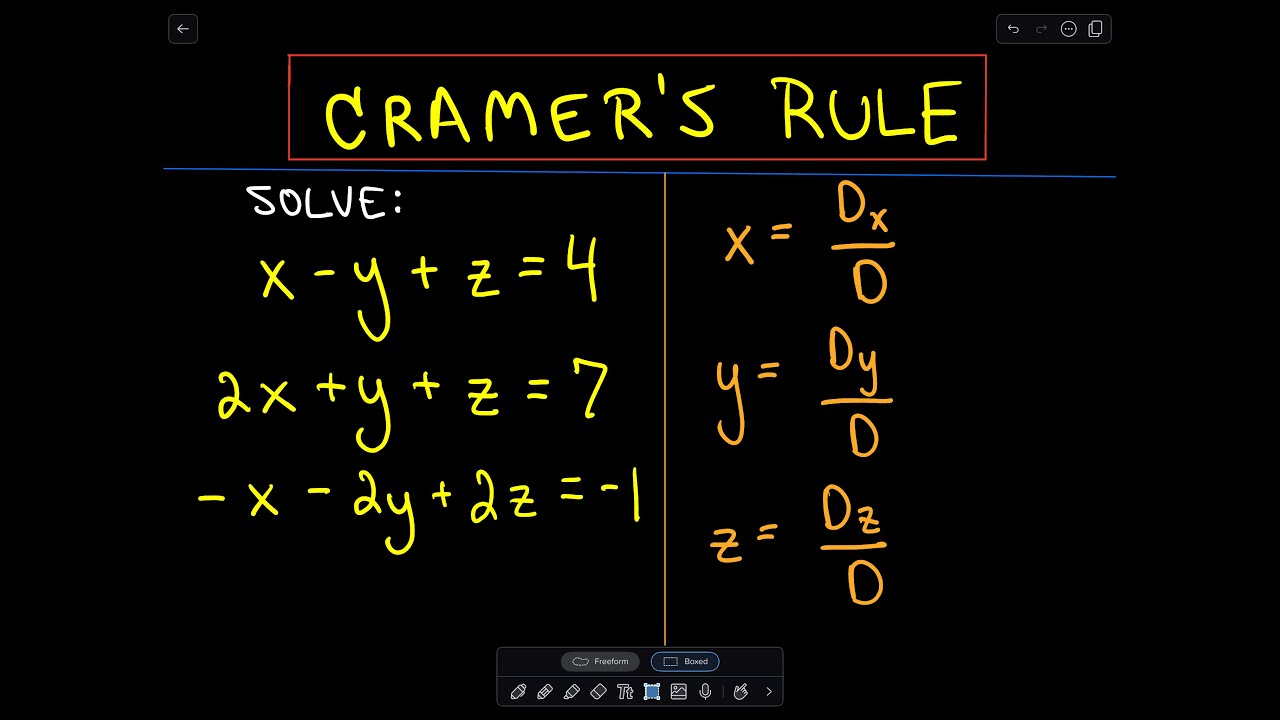
Cramer S Rule To Solve A System Of 3 Linear Equations Example 2 Youtube

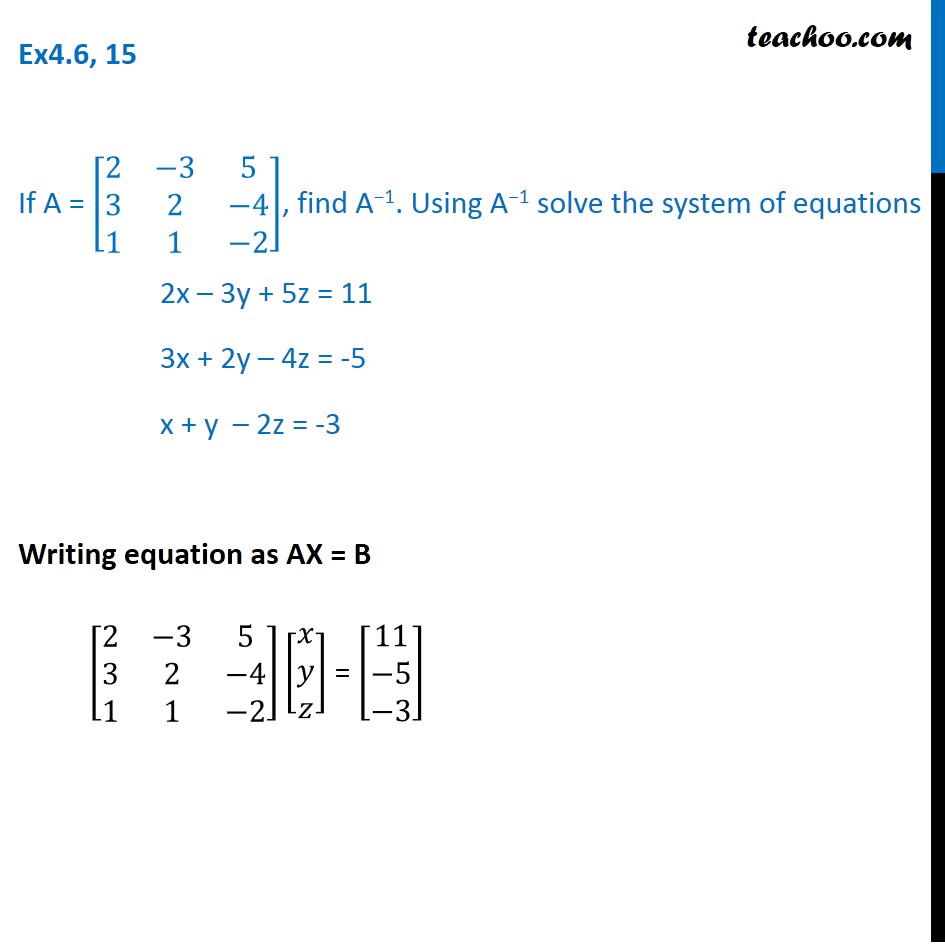
Ex 4 6 15 If A 2 3 5 3 2 4 1 1 2 Find A

2x y = 72 Here is the graph of 4x – 3y = 12 for values of x from 0 to 4 By drawing a second graph on the grid, work out an approximate solution to the simultaneous equations 4x – 3y = 12 and 3x 2y = 6 3 3 Solve algebraically the simultaneous equations x2 y2 = 25 y − 2x = 5 5

2 X 2 3y 1 6 And 3 X 2 Y 0 Problem Set 1 Q6 1 Of Linear Equation In Two Variables Youtube

How To Solve The Simultaneous Equation Xy 2 2log X 1 Log Y Quora

X 2 Y 2 74 And Xy 35 Find X Y And X Y 2x 3y 14 And Xy 8 Find 4x 2 9y 2 Youtube

Maths Guru Please Help Me Solve This Simultaneous Equations For My Young Photo Education 2 Nigeria

What Are The Simultaneous Equations Y 2x 1 Y 2 2x2 X Quora

Omtex Classes 3x Y 2 2x Y 3 Solve The Following Simultaneous Equations Graphically

New Syllabus Additional Mathematics Pages 51 100 Flip Pdf Download Fliphtml5

Graphically Solve The Following Pair Of Equations 2x Y 6 And 2x Y 2 0 Find The Ratio Of The Areas Youtube

1

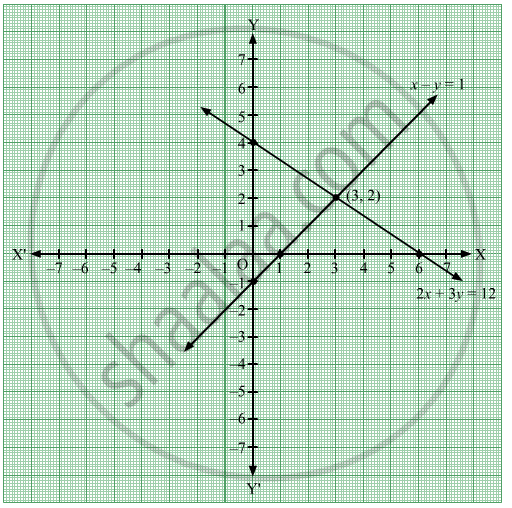
Solve The Following Simultaneous Equation Graphically 2x 3y 12 X Y 1 Algebra Shaalaa Com

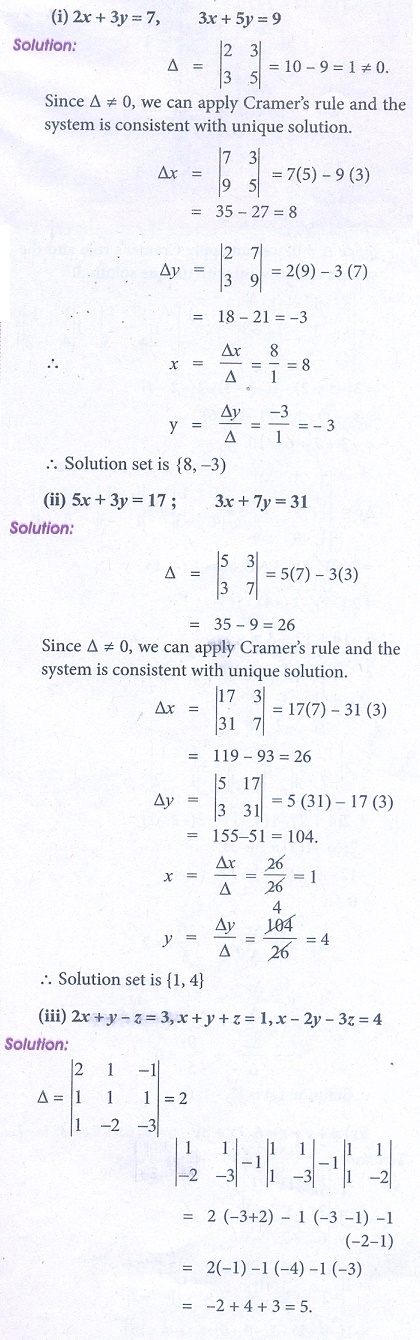
Exercise 1 2 Cramer S Rule Problem Questions With Answer Solution

Hw Extra Credit Problems

1 Simultaneous Equations Menu Algebra Menu Ducks Sheep Linear Type 4x 2y Teacher Examples 4x Y 8 Linear Type 4x 2y Questions 4x Ppt Download

Simultaneous Equations Gmat Math Study Guide

Chapter 13 Solving Simultaneous Equations

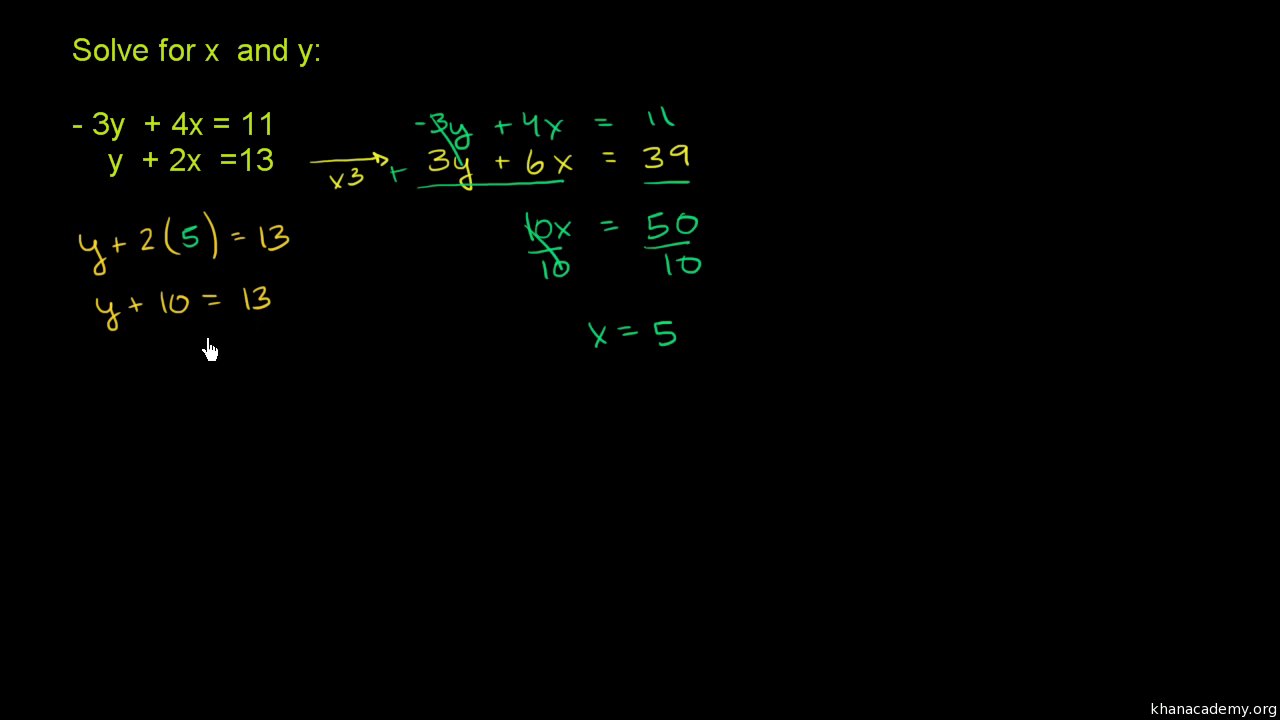
Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy

最も欲しかった 2x 3y 2 X Y 2 1 2 6 2x 3y 2 X Y 2 1 2

119 Ismsec1 Pdf Slope Square Root

How To Solve The Simultaneous Equation Xy 2 2log X 1 Log Y Quora

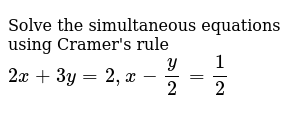
2x 3y 2 X Y 2 1 2 By Cremers Rule

2

Example 28 Solve By Matrix Method 3x 2y 3z 8 2x Y Z 1 Examples

Rd Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2

Simultaneous Equations 1 Ppt Download

Who Can Solve This X Y 15 And X Y 3 Find X And Y Quora

2x 3y 2 X Y 2 1 2 Practice Set 1 3 Crammers Method क र मरच पद धत Crammers Rule Grade 10 Youtube

Solve The Following Simultaneous Equations Using Cramer S Rule I 3x 4y 10 4x 3y 5 Sarthaks Econnect Largest Online Education Community

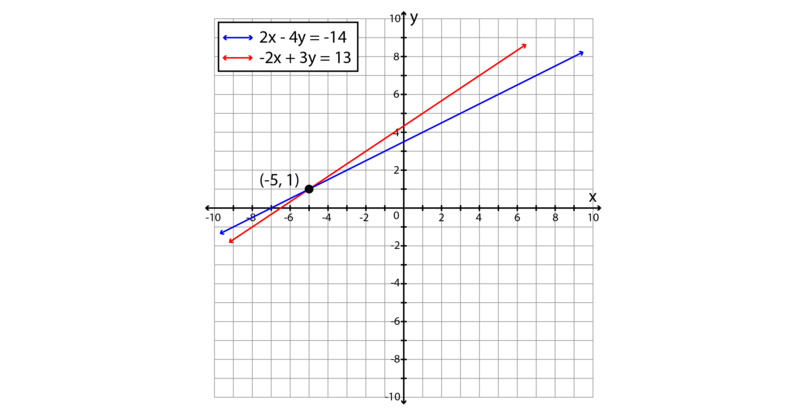
6 1 Tables And Graphs Simultaneous Linear Equations Siyavula

Q16 Answers Paper 2 June 18 Edexcel Gcse Maths Higher Elevise

How To Solve The Simultaneous Equation Xy 2 2log X 1 Log Y Quora

Maths Guru Please Help Me Solve This Simultaneous Equations For My Young Photo Education 2 Nigeria

X Y 1 2 Is The Solution Activity 1 To Solve The Simultaneous Equations By Determinant Method In The Blanks Y 2x 19 0 2x

2

Ex 3 3 2 Solve 2x 3y 11 And 2x 4y 24 And Hence Find M For

What Is Distance Of The Point 2 3 From The Line X Y 5 Quora

2x 3y 2 X Y 2 1 2 Practice Set 1 3 Crammers Method क र मरच पद धत Crammers Rule Grade 10 Youtube

Jpirasutog1blwh 最も好ましい X Y 2 X Y 2 Is Equal To X Y Z 0 Then X 2 Xy Y 2 Is Equal To

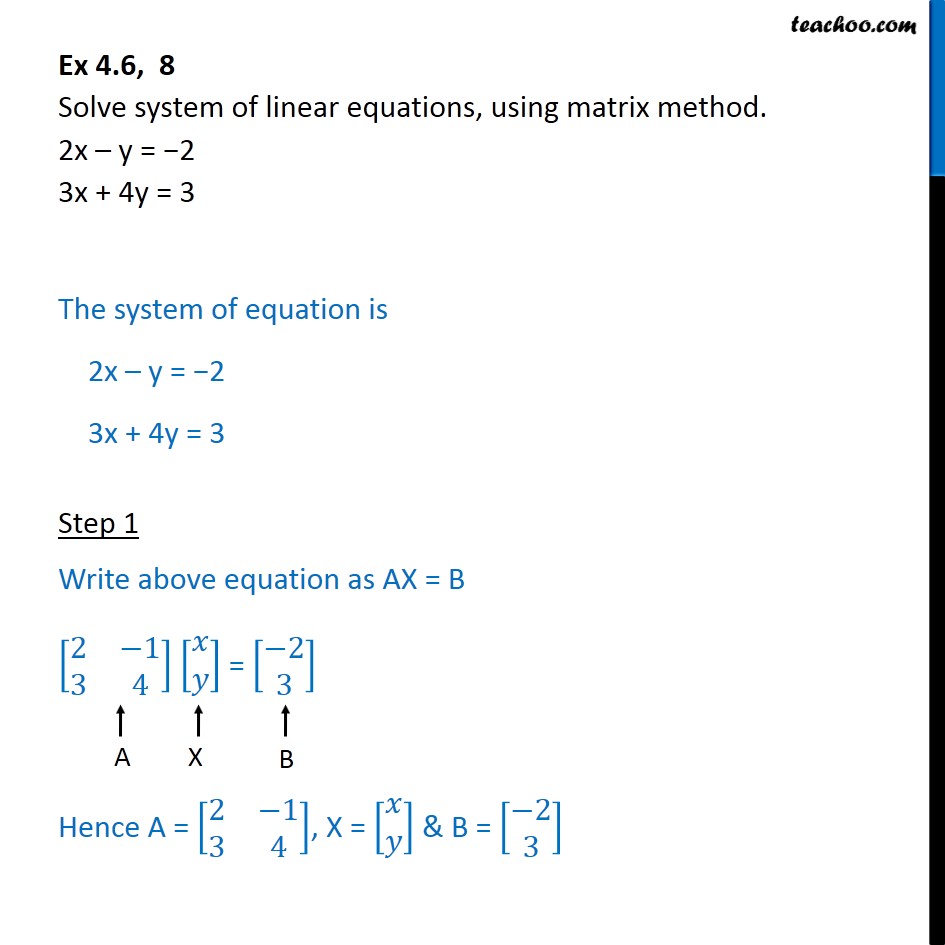
Ex 4 6 8 Solve Using Matrix Method 2x Y 2 3x 4y 3

Graph Graph Inequalities With Step By Step Math Problem Solver

1 Systemsofequationsandinequal Ities X 5 Y 25 25 5 Figure1 Consistent X Acirc Circ Rsquo 3y Acirc

Model Answers To Hwk 5 1

2x 3y 2 X Y 2 1 2 Using Cramer S Rule Brainly In

If 2x Y 2 5 3x Y 1 3 2 X 2y 1 6 Then

2

4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula

Simultaneous Equation 4 Youtube

How To Solve This Linear Equation Using The Gauss Jordan Elimination Method 2x 5y 3z 8 4x 3y 5 4x Y 2z 2 Quora

2x 3y 2 X Y 2 1 2 Using Cramers Rule Brainly In

Jpirasutog1blwh 最も好ましい X Y 2 X Y 2 Is Equal To X Y Z 0 Then X 2 Xy Y 2 Is Equal To

Graph Graph Inequalities With Step By Step Math Problem Solver

Graph Graph Inequalities With Step By Step Math Problem Solver

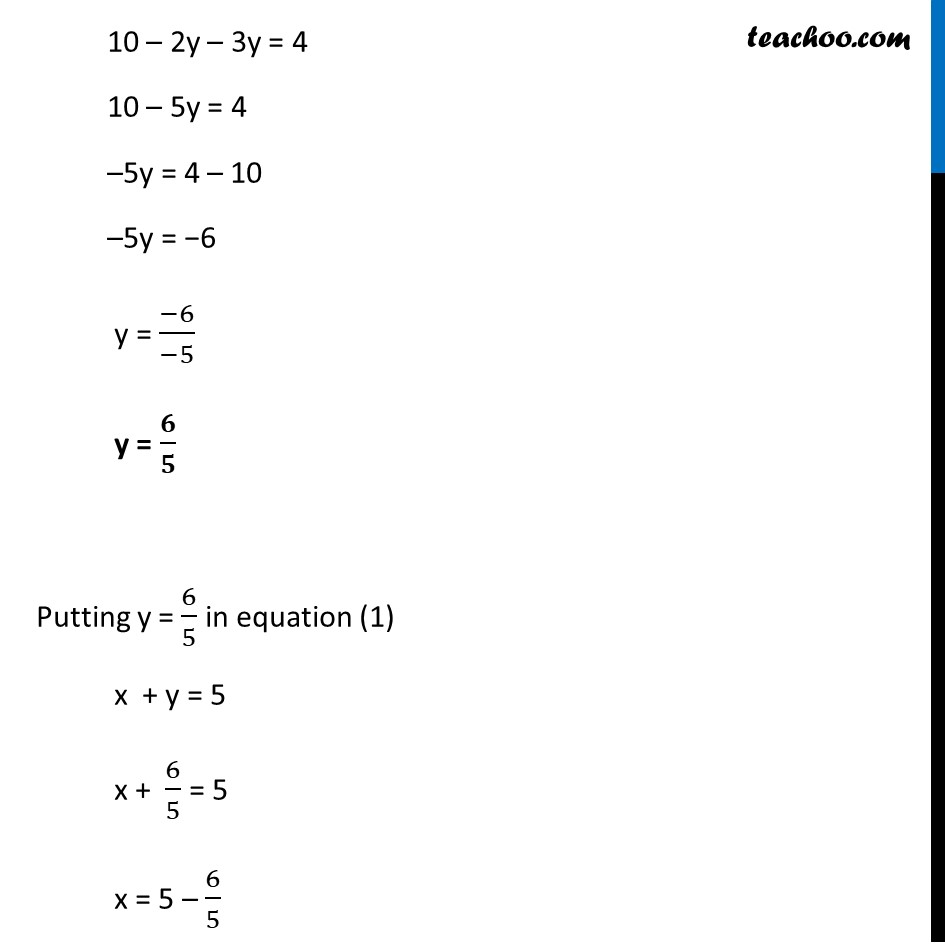
Ex 3 4 1 I Class 10 Solve By Elimination And Substitution

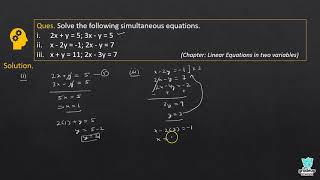
Q2 Solve The Following Simultaneous Equations I 2x Y 5 3x Y 5 Ii X 2y 1

Graph Graph Inequalities With Step By Step Math Problem Solver

Using Matrices Solve The Following System Of Equations 2x 3y 5z 11 3x 2y 4z 5 X Y 2z 3 Quora

Solve Simultenous Equations Using Cramer S Rule 2x 3y 2 X Y 2 1 2 Brainly In

Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook

If 2x 3y 14 And 2x 3y 2 Find The Value Of Xy

Solve The Following Simultaneous Equation Using Cramer S Rule 2x 3y 2 X Y 2 1 2 Brainly In

2

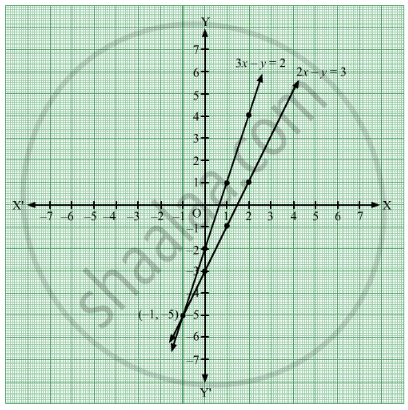
Solve The Following Simultaneous Equations Graphically 3x Y 2 2x Y 3 Algebra Shaalaa Com

2

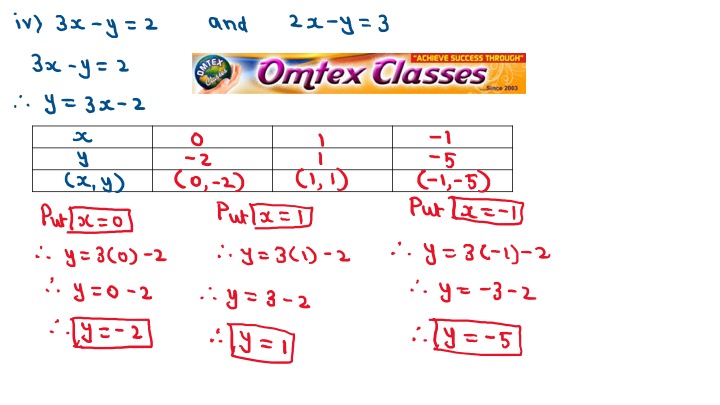
Omtex Classes 3x Y 2 2x Y 3 Solve The Following Simultaneous Equations Graphically

1

Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook

Q16 Answers Paper 2 June 18 Edexcel Gcse Maths Higher Elevise

Graph Graph Inequalities With Step By Step Math Problem Solver

Solve The Following Simultaneous Equations Graphically I 3x Y 2 2x Y 3 Ii 3x 4y 7 5x 2y 0 Iii 2x 3y 4 3y X 4 Sarthaks Econnect Largest Online Education Community

Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook

Solve The Following System Of Equations By Using Matrix Inversion Method 2x Y 3z 9 X Y Z 6 X Y Z 2